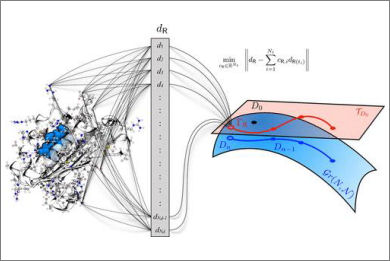
Born–Oppenheimer molecular dynamics (BOMD) is a powerful but expensive technique. The main bottleneck in a density functional theory BOMD calculation is the solution to the Kohn–Sham (KS) equations that requires an iterative procedure that starts from a guess for the density matrix. Converged densities from previous points in the trajectory can be used to extrapolate a new guess; however, the nonlinear constraint that an idempotent density needs to satisfy makes the direct use of standard linear extrapolation techniques not possible. In this contribution, we introduce a locally bijective map between the manifold where the density is defined and its tangent space so that linear extrapolation can be performed in a vector space while, at the same time, retaining the correct physical properties of the extrapolated density using molecular descriptors. We apply the method to real-life, multiscale, polarizable QM/MM BOMD simulations, showing that sizeable performance gains can be achieved, especially when a tighter convergence to the KS equations is required.
Polack, É.; Dusson, G.; Stamm, B. & Lipparini, F.
J. Chem. Theory Comput. 17, 6965-6973 (2021) https://doi.org/10.1021/acs.jctc.1c00751